To address the problem, we first geometrically define the concept of difference subspace (DS), which represents the difference components between two subspaces; these components are based on the canonical angles. A DS is a natural extension of the difference vector concept to a pair of subspaces.
Q. Why is the union of two subspaces not a subspace?
The union of two subspaces is a subspace if and only if one of the subspaces is contained in the other. Then I claim the x+y can’t be in either subspace, hence, can’t be in their union; hence, the union is not closed under addition, so it’s not a subspace.
Table of Contents
- Q. Why is the union of two subspaces not a subspace?
- Q. Is intersection of two subspaces a subspace?
- Q. How do you prove subspaces?
- Q. How do you know if its a subspace?
- Q. Are all subsets subspaces?
- Q. Is 0 vector a subspace?
- Q. Does a subspace have to contain the zero vector?
- Q. Why must every subspace contain the zero vector?
- Q. Can a subspace be empty?
- Q. How do you prove a subspace is non empty?
- Q. How do you prove a set is non empty?
- Q. Is the zero vector span?
- Q. Can a span be an empty set?
- Q. Can a basis be empty?
- Q. How do you know if a vector is a basis?
- Q. Is the basis of a vector space unique?
- Q. What is a span of a vector?
Q. Is intersection of two subspaces a subspace?
The intersection of two subspaces V, W of R^n IS always a subspace. Note that since 0 is in both V, W it is in their intersection. Second, note that if z, z’ are two vectors that are in the intersection then their sum is in V (because V is a subspace and so closed under addition) and their sum is in W, similarly.
Q. How do you prove subspaces?
To show a subset is a subspace, you need to show three things:
- Show it is closed under addition.
- Show it is closed under scalar multiplication.
- Show that the vector 0 is in the subset.
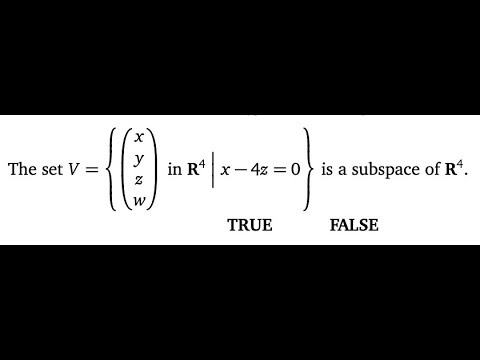
Q. How do you know if its a subspace?
In other words, to test if a set is a subspace of a Vector Space, you only need to check if it closed under addition and scalar multiplication. Easy! ex. Test whether or not the plane 2x + 4y + 3z = 0 is a subspace of R3.
Q. Are all subsets subspaces?
Since a vector space requires that any linear combination of two or more of its elements is also one of its elements (this is termed closure), is not a vector space, and hence is not a subspace of . In short, therefore, a subset of a vector space is termed a subspace if whenever and are in .
Q. Is 0 vector a subspace?
3 Answers. Yes the set containing only the zero vector is a subspace of Rn. It can arise in many ways by operations that always produce subspaces, like taking intersections of subspaces or the kernel of a linear map.
Q. Does a subspace have to contain the zero vector?
The formal definition of a subspace is as follows: It must contain the zero-vector. It must be closed under addition: if v1∈S v 1 ∈ S and v2∈S v 2 ∈ S for any v1,v2 v 1 , v 2 , then it must be true that (v1+v2)∈S ( v 1 + v 2 ) ∈ S or else S is not a subspace.
Q. Why must every subspace contain the zero vector?
Every vector space, and hence, every subspace of a vector space, contains the zero vector (by definition), and every subspace therefore has at least one subspace: It is closed under vector addition (with itself), and it is closed under scalar multiplication: any scalar times the zero vector is the zero vector.
Q. Can a subspace be empty?
1 Answer. The answer is no. The empty set is empty in the sense that it does not contain any elements. Thus the zero vector is not a member of the empty set.
Q. How do you prove a subspace is non empty?
A subset U of a vector space V is called a subspace, if it is non-empty and for any u, v ∈ U and any number c the vectors u + v and cu are are also in U (i.e. U is closed under addition and scalar multiplication in V ).
Q. How do you prove a set is non empty?
Well, in general if you want to prove that a set S is not empty, then you just have to prove that it contains an element. This element can be the 0 element or any other (this don’t matter). Now, suppose that V is a F vector space, W⊂V, v+w∈W for every v,w∈W and αu∈W for every u∈W and every α∈F.
Q. Is the zero vector span?
Because a linear combination with arbitrary scalars of no vectors yields zero vectors,the result of such a sum is the zero scalar. Therefore the components of any vector spanned by the empty set are 0 and the only vector this is true of is 0. Therefore, the empty set spans {0}.
Q. Can a span be an empty set?
In the context of vector spaces, the span of an empty set is defined to be the vector space consisting of just the zero vector. This definition is sometimes needed for technical reasons to simplify exposition in certain proofs.
Q. Can a basis be empty?
A basis is a collection of vectors that is linearly independent and spans the entire space. Thus the empty set is basis, since it is trivially linearly independent and spans the entire space (the empty sum over no vectors is zero).
Q. How do you know if a vector is a basis?
The criteria for linear dependence is that there exist other, nontrivial solutions. Another way to check for linear independence is simply to stack the vectors into a square matrix and find its determinant – if it is 0, they are dependent, otherwise they are independent.
Q. Is the basis of a vector space unique?
That is, the choice of basis vectors for a given space is not unique, but the number of basis vectors is unique. This fact permits the following notion to be well defined: The number of vectors in a basis for a vector space V ⊆ R n is called the dimension of V, denoted dim V.
Q. What is a span of a vector?
The span of a set of vectors is the set of all linear combinations of the vectors. For example, if and. then the span of v1 and v2 is the set of all vectors of the form sv1+tv2 for some scalars s and t.